
The Traffic Accident Reconstruction Origin -Article-


The Traffic Accident Reconstruction Origin -Article-
|

|
Pedestrian Accident Reconstruction:Review and Update
by Luis Martinez
Abstract
Pedestrian accident reconstruction has become a critical, important aspect of the field of motor vehicle collision reconstruction. The dynamics, methodology, and principles involved are somewhat different than those routinely used to reconstruct vehicle collisions. The field is one quickly becoming highly specialized. This paper presents a background of the problem, a limited summary of the work available in the field, and discusses the current reconstruction methodologies as they refer to pedestrian walking speeds, impact orientation, and determining impact speeds.
The Pedestrian Problem
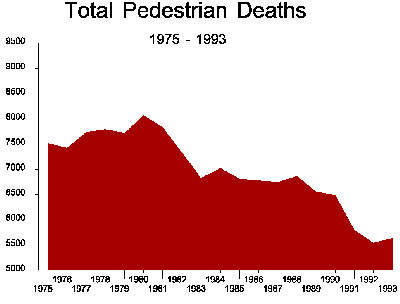 In the United States alone approximately 7,000 pedestrians are killed as a
result of motor vehicle crashes every year, and approximately 119,000 are injured.
Table1
shows a breakdown of yearly pedestrian death figures, since 1975, separated by gender.
This data was obtained from the U.S. Department of Transportation's Fatal Accident
Reporting System (FARS).
In the United States alone approximately 7,000 pedestrians are killed as a
result of motor vehicle crashes every year, and approximately 119,000 are injured.
Table1
shows a breakdown of yearly pedestrian death figures, since 1975, separated by gender.
This data was obtained from the U.S. Department of Transportation's Fatal Accident
Reporting System (FARS).
During the 19-year period from 1975 through 1993 pedestrian fatalities peaked at 8,070 deaths in 1980, and appear to be steadily declining ever since. The Table to the left depicts the data in table I using a line-area graph.
Since 1975, between 14 and 17 percent of motor vehicle deaths have been pedestrians. Pedestrians accidents are exceeded only by falls and motor vehicle accidents as a cause of accidental deaths. The annual cost of pedestrians accidents to society exceed one billion dollars.
Once the pedestrian statistical picture is examined closely, it has been determined that older adults and young children face the greater risk on our roadways. Pedestrian death rates among older adults have been decreasing since 1950, but people 65 years of age and older have the highest pedestrian death rates.
This rate is more than twice as high as it is for younger people. Thirty-three percent of all motor vehicle deaths of 1-9 year old victims are pedestrians. Sixty-six percent of pedestrian deaths among children younger than 13 occur between 3 and 9 pm. A peak in pedestrian deaths is usually reached between 6 pm to 9 pm. This is no doubt partially the result of pedestrians being struck on high speed roadways during hours of darkness.
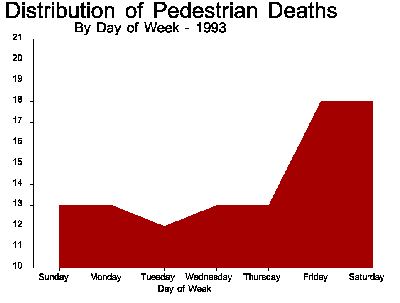
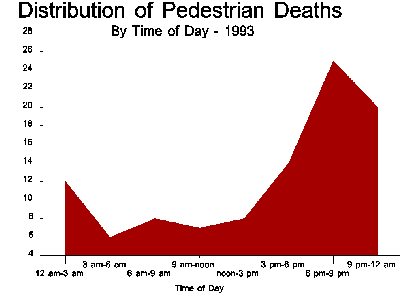 TableII is a summary of the distribution of
pedestrian deaths during 1993 by time of day, and TableIII
shows the deaths according to
the day of the week. The same information is also presented in an line-area graph format,
in the figures to the left. Fatalities appeared to be over represented during Fridays and
Saturdays.
TableII is a summary of the distribution of
pedestrian deaths during 1993 by time of day, and TableIII
shows the deaths according to
the day of the week. The same information is also presented in an line-area graph format,
in the figures to the left. Fatalities appeared to be over represented during Fridays and
Saturdays.
Sixty percent of pedestrians 16 years and older killed in night time crashes have very high blood alcohol concentrations (0.10 percent or more). Thirty-four percent have no alcohol in their blood. Among 16-20 year old, forty-two percent have blood alcohol concentrations of 0.10 percent or more.
The percentage of pedestrians 16 years and older with blood alcohol concentrations of 0.10 y percent or more who were killed in night time crashes remained about the same (about 60 percent) between 1980 and 1993, while the percentage of fatally injured passenger vehicle drivers with blood alcohol concentrations of 0.10 percent or more decreased by 16 percent during the same period. This may be the net effect of active drunk driver campaigns by law enforcement and the media in general. There has been an active renewed interest in walking as a form of exercise and transportation in recent years. This increase in pedestrian traffic along with an ever-increasing traffic volume has resulted in increased sharing of trafficways by vehicles and pedestrians alike.
Pedestrian accident reconstruction
A collision involving the death of a pedestrian can be one of the most difficult, yet rewarding, tasks facing any reconstructionist today. There are many issues facing the investigator(s) for which the answers are not easy to reach.
Pedestrian Walking speed
 One of the issues critical to the investigation, particularly when attempting to
determine time and distance problems, is that of pedestrian speed. Next to
perception/reaction time, there is probably no other area of collision investigation
where there is such a disparity on what a specific value ought to be. Well known
publications have established specific walking speeds, primarily for purposes of
highway design and sign placement, at 4 feet per second and from 2.5 to 6 feet per
second. In addition, many other publications and technical papers carry tables on
pedestrian walking velocity based on empirical data. TableIV
is such an example,
obtained from Jerry Eubanks recently published textbook on pedestrian accident
reconstruction. The figure above presents the same data in a bar graph format.
One of the issues critical to the investigation, particularly when attempting to
determine time and distance problems, is that of pedestrian speed. Next to
perception/reaction time, there is probably no other area of collision investigation
where there is such a disparity on what a specific value ought to be. Well known
publications have established specific walking speeds, primarily for purposes of
highway design and sign placement, at 4 feet per second and from 2.5 to 6 feet per
second. In addition, many other publications and technical papers carry tables on
pedestrian walking velocity based on empirical data. TableIV
is such an example,
obtained from Jerry Eubanks recently published textbook on pedestrian accident
reconstruction. The figure above presents the same data in a bar graph format.
Eubanks concluded that men under 55 years of age have a walking speed of 5.4 feet per second while those over 55 slow to 5.0 feet per second. Women generally walk slower than men and those under 50 years of age have a walking speed of 4.5 feet per second, women over 50 walk at about 4.3 fps. According to Eubanks, women with small children walk at about 2.3 feet per second. He recommends continued testing and observation of pedestrian behavior under various circumstances.
A 1989 paper by S.J. Ashton included results of research performed in the United Kingdom in 1965, presented here as TableV. The results of this testing is very similar to those presented in the earlier table.
Pedestrian impact orientation
Another critical issue to be determined and researched on every pedestrian collision is that of pedestrian impact kinematics, or how the pedestrian moved at and through the impact phase of the collision event. Ravani classified the different pedestrian impact orientations into five distinctive groups (wrap, forward projection, fender vault, roof vault, somersault). These five distinctive styles of pedestrian kinematics have become defacto standards when describing impact dynamics. Understanding these impact orientations in relation to the vehicle position helps the investigator in determining how injury causation occurred.

In the wrap trajectory, the pedestrian is struck in the lower legs by the front of a decelerating vehicle. The striking portion of the vehicle must be lower than the height of the pedestrian. Upon impact the legs buckle and the torso bends over the hood and the chest impacts the top of the hood. The head impacts the hood in a whipping motion. After initial impact, the pedestrian tends to stay on the hood of the car and rides to a stop, sometimes sliding off the hood at stop.
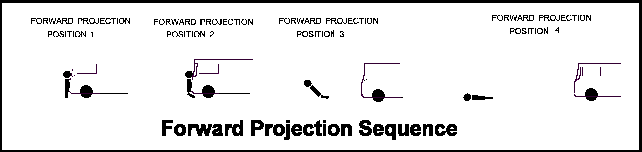
The next impact orientation is the forward projection. In this configuration the pedestrian is struck by a flat faced vehicle, such as a truck or van, and the force applied is well above the center of gravity of the pedestrian. This can also occurred when passenger vehicles strike small children. The pedestrian is quickly accelerated to the speed of the striking vehicle and then drops to the roadway surface ahead of the vehicle .

The fender vault involves pedestrians struck near a front corner of the vehicle. First contact is usually made at the legs, with the torso pivoting towards the hood. Due to the position of the pedestrian (near the vehicle's edge) he falls off the edge and does not impact the hood, striking the roadway. The pedestrians head may or may not impact the vehicle.
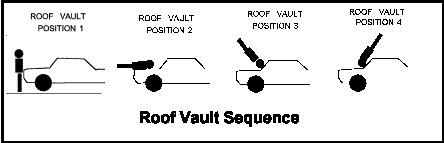
The fourth impact orientation is the roof vault, which begins initially like a wrap trajectory but in this case the pedestrian's legs do not stay ahead of the vehicle. Due to the impact forces the legs continue to rotate upward, with the pedestrian essentially standing on his head on or near the roof line. The vault maneuver is completed when the pedestrian leaves the vehicle, over the roof, and tumbles to the ground.
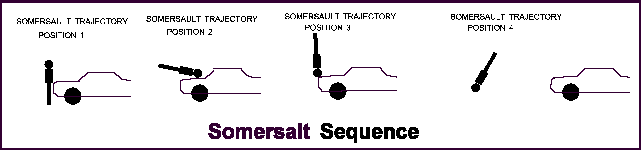
The last impact orientation is the somersault, which is similar in its initiation to the roof vault. During a somersault the vehicle is typically decelerating at impact and this causes the pedestrian to be thrown ahead of the vehicle. One would expect serious or even fatal head injuries as a result of this impact type. The impact orientations discussed here are applicable primarily to adult pedestrians. They may not always be applicable to small children due to their height
Impact speeds
The subject of impact speeds is one of common importance to investigators of pedestrian collisions, particularly with those personnel tasked with determining violations of the law. Estimating vehicle speeds, as it relates to negligence and civil liability, is also crucial in civil cases. There are a number of different approaches to the speed question when dealing with pedestrian impacts. Ashton presented the various available techniques in descending order of accuracy, although the order can certainly be subject to interpretation:
1. Skid Mmarks;
2. Pedestrian Throw Ddistance;
3. Vehicle Damage;
4. Pedestrian Injury;
5. Witness/Ddriver Sstatements.
From skid marks
The easiest and most commonly used method for determining the speed of a vehicle striking a pedestrian is by using skid marks. of course, this presumes the striking vehicle was braking and the total distance the vehicle skidded to a stop can be estimated. There will be some speed loss as a result of the impact with the pedestrian but this is an insignificant loss, around 1-2 miles per hour in most cases due the large difference in mass between the vehicle and pedestrian. The standard dissipation of energy equation, in one of its various forms, can be used to determine the vehicle's initial speed:
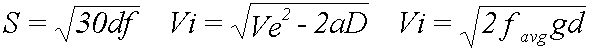
From pedestrian throw distance
The second best alternative to the speed question is by using the pedestrian's total throw distance to estimate an impact speed for the striking vehicle. Many times striking vehicles do not brake and throw distance is the only available evidence to estimate impact speed. When a pedestrian is struck by a moving vehicle he is accelerated in the direction of the velocity vector of the striking vehicle. The distance that the body is thrown forwards is an indicator of the speed of the vehicle at impact.
A simple and better known approach to estimate impact speed of the striking vehicle is to use the sliding distance the pedestrian body skidded to a stop (not throw distance) and apply an energy dissipation equation. This is an easily defended approach but the investigator must determine the first impact point:
Where: S=pedestrian speed after impact,mph
d=sliding distance of body
f=coefficient of friction for sliding body
This method is a "safe" tactic to use when the pedestrian's first impact point along the roadway can be established, as the speed calculated will logically represent only a fraction of the vehicle's impact speed, that is, it will be on the "low" side.
Some of the earliest work along the lines of calculating speed from throw distance was done by H. Appel in 1975, and G. Sturtz in 1976. Appel deduced that throw distance increased as the square of the impact speed. This was as a result of an analysis of 137 real accidents in which the victims were struck directly by the front of the striking vehicle. His analysis further noted that children tended to be thrown further than adults, and that pedestrians struck by high fronted vehicles tended to be thrown further than those struck by low fronted vehicles. TableVI details Appel's results.
Later analysis by Sturtz of essentially the same data as Appel, yielded a slightly different result. Sturtz found that a linear plus cubic method produced the best fit. His results are presented in TableVII.
Schmidt and Nagel also developed an equation to relate impact speed to throw distance. However, their approach required the user to know the maximum projectile height of the center of mass. This is not something the investigator routinely knows, or can easily determine outside the testing lab.
Another approach to the speed from throw distance problem was first presented in a 1983 paper by Searle and Searle. They derived a formula that included the total trajectory of the pedestrian after impact, including the bouncing and sliding after it first contacts the road surface:
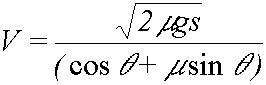
Where: V=launch velocity, fps
q =launch angle
F =acceleration due to gravity, 32.2
s=throw distance, ft
Since the angle of projection or launch is usually not known, Searle & Searle derived a set of equations that gives upper and lower bounds by considering those values of the projection angle that will maximize and minimize the expression:
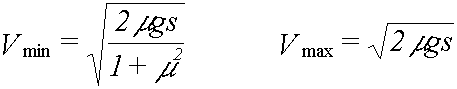
Pedestrian coefficient of friction is another area of considerable debate within the accident reconstruction, and a critical part of any throw distance formula. Searle & Searle reported friction coefficients of .66 on asphalt and .79 on grass, regardless of whether the surface is wet or dry, and these are the values they used in their equation.
Another speed-from-throw-distance equation making the rounds in this field can be found in Dr. Rudolf Limpert's 1989 book. Limpert's formula is based on data from test impacts where the vehicle was braking during and after the impact:
Where: Vc=collision speed, mph
a=vehicle acceleration, in g-units
S=throw distance, ft
In Nortwestern's 1990 reconstruction textbook, an alternate method to determine speed from throw distance was discussed by Fricke, to be used with large trucks, vans, and other blunt front end vehicles. He used a fall velocity equation to calculate an initial vehicle velocity when the total throw distance is known but the first touchdown location of the pedestrian is not known. To utilize Fricke's method, the fall distance must be calculated first, then either the fall equation and the slide-to-stop equation can be solved for initial vehicle velocity:
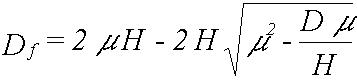
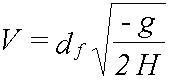
Where: Df =fall distance
F =body drag factor
H=vertical distance body center of mass fell
ds=horizontal distance body traveled while sliding
g=32.2
d=total distance from impact to final rest
Finally, an excellent textbook on pedestrian accident reconstruction, published in 1994 by Jerry Eubanks, proposed a quadratic equation approach to solving the speed from throw distance problem. Eubanks' approach requires some additional information over other methods. Investigators wishing to utilize this technique should review the discussion in his book. A quadratic equation is a formula where there are two unknown values, but can be solved using A, B, and C coefficients:
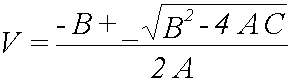
The values to be applied to the above quadratic equation, to replace the A-B-C parameters, are:
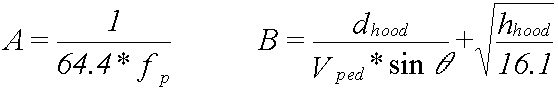
Where:
fp= coefficient of friction of pedestrian
q = angle between vehicle's pre-impact path and pedestrian's direction of travel
dhood= the distance the point of initial contact on the vehicle to the side of the vehicle the pedestrian exits
Vped= velocity of pedestrian pre-impact
hhood= highest point of contact on vehicle
dt= throw distance From vehicle damage/pedestrian injury
From Vehicle Damage
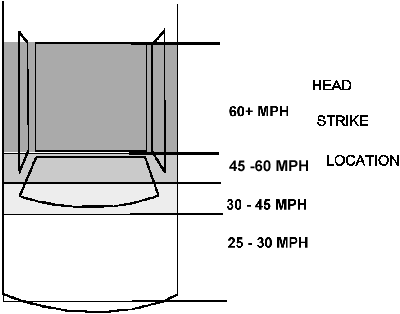
Vehicle damage is another, albeit less reliable, method for estimating impact speed. The higher the impact speed of the vehicle the further back from the front end of the vehicle the damage will tend to be, and the more severe. This is a general rule and, as all such rules, should be applied judiciously and with massive amounts of common sense. The pedestrian's position at impact, his position in relation to the front of the vehicle, as well as the height of the pedestrian are factors that will influence the location of impacts.
During the author's attendance at the Institute of Police Technology and Management's Pedestrian Accidents and Human Factors course on September 1993, information was provided on the correlation of head strike location and vehicle impact speeds. Prospective users of this information are cautioned that in in-line collisions (pedestrian facing directly at or directly away from vehicle) bodies tend to "sit" on the vehicle's hood and slide towards the windshield. No referential or empirical information was provided during the course to validate this approach.
The author's research and review of two SAE papers,, where both dummies and cadavers were used in a laboratory setting to replicate real-world pedestrian accidents, would seem to validate the correlation to a very limited degree. In order to fully validate this approach expanded testing would be required.
From Pedestrian Injury
The area of correlating pedestrian injury to vehicle impact speed, although researched in the past, is somewhat imprecise. Fatalities have been noted at relatively low speeds, with minor injuries at high speeds. Thus, determining speed from injuries is highly speculative and not as reliable as the other methods described above.
Witness and Driver Statements
Determining impact speeds from witness and driver statements is notoriously unreliable for many obvious reasons.
Conclusions
The investigation and reconstruction of pedestrian traffic collision in the United States has progressed to a point where it now stands on its own as a specialized field within collision investigation. Although the number of pedestrian deaths appears to be at its lowest level and decreasing annually, continued research and analysis of these types of collisions is crucial. Essential to a complete reconstruction of a pedestrian collision are the issues of pedestrian walking speed, impact orientation, and impact speeds. A thorough investigation must address these in order to be able to answer all necessary questions, or as many as possible with available data, arising out of a pedestrian collision.
Luis Martinez is a Sergeant with the Eloy (AZ) Police Department and teaches accident investigation and criminal justice courses at Central Arizona College. He holds a B.A. in management and is nearing completion of a Master Degree in Education at Northern Arizona University. Luis holds ACTAR accreditation number 38. Sgt. Luis Martinez can be reached at luisem@primenet.com
REFERENCES
1 "Fatality Facts 1994", Insurance Institute for Highway Safety, 1994.
2 "Manual On Uniform Traffic Control Devices", Federal Highway Administration, U.S. Department of Transportation, 1988.
3 "A Policy On Geometric Design Of Highways And Streets", American Association of State Highway and Transportation Officials, 1990.
4 Eubanks, J., "Pedestrian Accident Reconstruction", Tucson:Lawyers & Judges Publishing, 1994.
5 Ashton, S.J., "Pedestrian Accident Investigation and Reconstruction", Seminar on Special Problems in Traffic Accident Reconstruction, IPTM, 1989.
6 Ravani, B., Broughham, D., and Mason, R.T., "Pedestrian Post-Impact Kinematics and Injury Patterns", Society of Automotive Engineers Paper No.811024, 1981.
7 Guenther, D.A., Wiechel, J.F., "Review of Pedestrian Safety Research in the United States", Society of Automotive Engineers Paper No.890757, 1989.
8 Appel, H., Sturtz, G., and Gotzen, L., "Influence of Impact Speed and Vehicle Parameters on Injuries to Children and Adults in Pedestrian Accidents", IRCOBI, 1975.
9 Schmidt, D.N., Nagel, D.A., "Pedestrian Impact Case Study", Proceedings 15th Conference, American Association for Automotive Medicine, 1971.
10 Searle, J.A., Searle, A., "The Trajectories of Pedestrians, Motorcycles, Motorcyclists, etc., Following a Road Accident", Society of Automotive Engineers Paper No.831622, 1983.
11 Limpert, R., "Motor Vehicle Accident Reconstruction and Cause Analysis", Charlottesville:The Michie Company, 1989.
12 Fricke, L.B., "Traffic Accident Reconstruction", Evanston:Northwestern University Traffic Institute, 1990.
13 Appel, H., Heger, A., "Reconstruction of Pedestrian Accidents with Dummies and Cadavers", Society of Automotive Engineers Paper No.806069, 1980.
14 Ashton, S.J., Cesari, D., and van Wijk, J., "Experimental Reconstruction and Mathematical Modelling of Real World Pedestrian Accidents", Society of Automotive Engineers Paper No.830189, 1983.
|
Copyright ©
|